
The discovery of irrational numbers came from a sect
Was a mathematician drowned by members of a strange cult? Many myths and legends revolve around the history of irrational numbers.
He was punished with death for his discovery of irrational numbers - at least that is the legend surrounding the ancient scholar Hippasos of Metapont. What actually happened in the 5th century BC is still unclear to this day.
Hippasus apparently belonged to the Pythagoreans, a sect that focussed on mathematics and number mysticism, among other things. A core element of their teachings related to harmonic numerical relationships, which included fractions of integers. From the perspective of the time, the whole world could be described using natural and rational numbers. However, when Hippasos examined the length ratios of a pentagram - the symbol of the Pythagoreans - he realised that some side lengths could not be expressed as fractions.
This was the first documented proof of the existence of irrational numbers. Some stories say that the Pythagoreans took offence at this, because such numbers violated their world view. In other interpretations, Hippasos had published his result and thus violated the confidentiality provisions of the covenant. Either way, Hippasos apparently drowned in the sea after his discovery. Sometimes it is reported that the Pythagoreans threw him off a ship after he informed them of his discovery - or that his death was an accident, but the Pythagoreans regarded it as a punishment from God. Current interpretations assume that none of this is true. On the contrary: Hippasus' discovery was already recognised at the time as a mathematical breakthrough that the Pythagoreans were proud of - provided that the proof actually came from Hippasus. Because not even that is certain.
The only thing that has been handed down is that Pythagoreans proved the incommensurability of certain quantities, from which the existence of irrational numbers follows. The Pythagoreans themselves are also the subject of a number of stories, the veracity of which is often questionable. The community was probably founded in what is now southern Italy by Pythagoras of Samos - the Greek scholar after whom the famous Pythagorean theorem is named (although it is unclear whether he actually proved the theorem).
The Pythagoreans rejected wealth and lived a vegetarian and ascetic lifestyle. They also believed in reincarnation, which set them apart from the rest of the ancient Greek population. In addition to mathematics, the community was probably concerned with philosophy and politics. However, their views were not very popular, which is why they were persecuted: There were several attacks on the community; a few decades after Pythagoras' death, the league disappeared completely.
Numbers beyond fractions
Although much about the Pythagoreans remains mysterious, they are widely regarded as the discoverers of irrational numbers. We now learn at school that there are values that cannot be expressed as the quotient of two whole numbers. However, this realisation does not seem at all natural: after all, all irrational values can be approximated exactly by fractions - even if it is more difficult with some irrational numbers than with others.
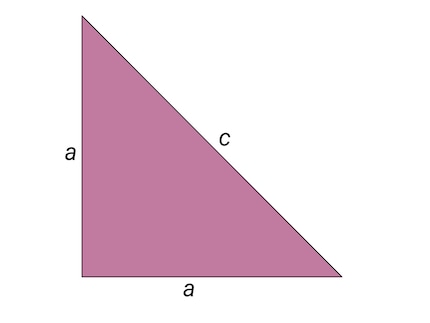
The proof presented by Hippasus (or another Pythagorean) is most easily illustrated with an isosceles and right-angled triangle, even though the original proof was probably performed on a different geometric figure (probably the pentagon). The two cathets of length a in an isosceles right-angled triangle therefore form a right angle, opposite which lies the hypotenuse of length c.
Source: © Spektrum der Wissenschaft / Manon Bischoff (detail)
Such a triangle has a fixed aspect ratio a⁄c. If both a and c are rational numbers, the lengths of the sides of the triangle can be chosen so that a and c correspond to the smallest possible natural numbers (i.e. they have no common divisors). For example, if the aspect ratio were ⅔, you would choose a = 2 and c = 3. This means: Assuming that the lengths of the triangle correspond to rational numbers, a and c are integers and have no common divisors.
A proof of contradiction
Hippasos used this fact to create a contradiction - and thus prove that the original assumption must be wrong. Firstly, he used the Pythagorean theorem to express the length of the hypotenuse c as a function of the two cathets a: 2a2 = c2. Since a and c are integers, it follows from the previous equation that c2 must be an even number. Accordingly, c is also divisible by two: c = 2n, where n is a natural number.
Substituting c = 2n into the original equation results in: 2a2 = (2n)2 = 4n2. The two can be reduced on both sides, which gives the following result: a2 = 2n2. Since a is also an integer, it follows that a2 and therefore also a are even numbers. However, this contradicts the original assumption; because if a and c are both even, they cannot be divisors.
This allowed Hippasos to conclude that the aspect ratio of an isosceles right-angled triangle a⁄c cannot correspond to a rational number. In other words, there are numbers that cannot be represented as the ratio of two integer values. For example, if you choose the lengths of the two sides of the triangle a = 1, then c = √2. And as we know today, √2 is an irrational number. Its decimal places continue indefinitely without ever repeating themselves.
From today's perspective, the existence of irrational values does not seem too surprising because we are confronted with this fact at a very young age. It is difficult to assess what effect such a realisation must have had around 2500 years ago. In any case, it must have turned the mathematical world view upside down. No wonder there are so many myths and legends about this discovery.
Spectrum of science
We are partners of Spektrum der Wissenschaft and want to make well-founded information more accessible to you. Follow Spektrum der Wissenschaft if you like the articles.
Original article on Spektrum.deExperts from science and research report on the latest findings in their fields – competent, authentic and comprehensible.